Effective Theory for the Measurement-Induced Phase Transition of Dirac Fermions

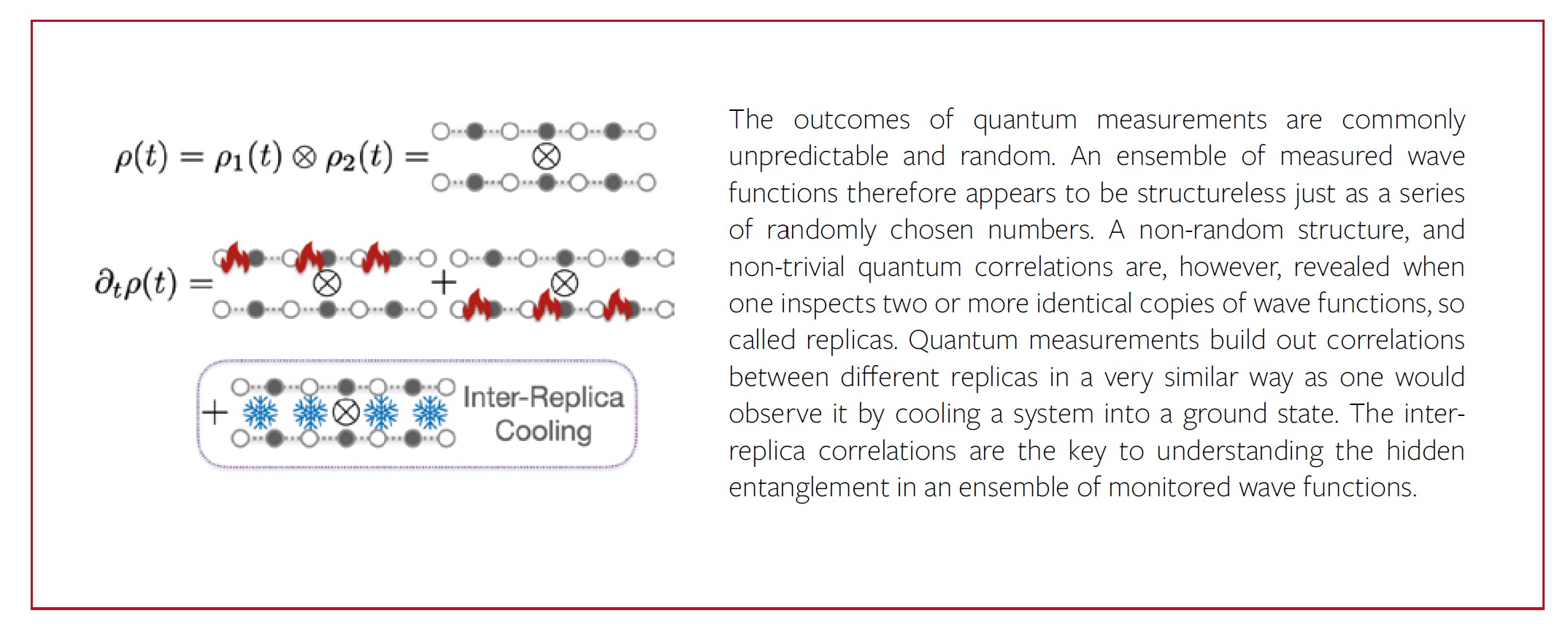
The recent discovery of a novel type of phase transition – driven by the competition between a quantum system’s internal evolution and its external observation – has sparked significant research into its nature and origin. Induced by measurements, the transition is marked by a qualitative change of the behavior of the entanglement entropy. Here, we provide the missing bridge from micro- to macrophysics by developing a new theory that describes the transition from the perspective of nonequilibrium quantum statistical mechanics.

The decisive step in understanding the change from microscopic simplicity to macroscopic complexity lies in the identification of the relevant degrees of freedom. Our
work takes that step for this novel class of measurement-induced phase transitions. Such transitions then appear as a natural consequence of an underlying quantum field theory, distilling the quantum physics of the monitored many-body wave function from the noisy background that is caused by the random measurement outcomes. To make this more concrete, we consider a system of free Dirac fermions and show that, by observation alone, the Dirac fermions are made to behave like strongly interacting electrons or planar magnets – they undergo what is known as a Berezinskii-Kosterlitz-Thouless quantum phase transition.

Our approach connects the novel class of measurement-induced transitions more firmly to quantum phase transitions. We demonstrate that measurement-induced
transitions reflect profound changes in many-body wave functions due to observation. In this picture, the newly discovered entanglement signatures just represent the
tip of the iceberg for quantum correlations in monitored many-body wave functions.

Publication: Effective Theory for the Measurement-Induced Phase Transition of Dirac Fermions, M. Buchhold, Y. Minoguchi, A. Altland, and S. Diehl, Phys. Rev. X 11, 041004